Lifting the Stone（求任意多邊形的重心）

Lifting the Stone

Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 65536/32768 K (Java/Others)
Total Submission(s): 5432 Accepted Submission(s): 2273


Problem Description There are many secret openings in the floor which are covered by a big heavy stone. When the stone is lifted up, a special mechanism detects this and activates poisoned arrows that are shot near the opening. The only possibility is to lift the stone very slowly and carefully. The ACM team must connect a rope to the stone and then lift it using a pulley. Moreover, the stone must be lifted all at once; no side can rise before another. So it is very important to find the centre of gravity and connect the rope exactly to that point. The stone has a polygonal shape and its height is the same throughout the whole polygonal area. Your task is to find the centre of gravity for the given polygon.

Input The input consists of T test cases. The number of them (T) is given on the first line of the input file. Each test case begins with a line containing a single integer N (3 <= N <= 1000000) indicating the number of points that form the polygon. This is followed by N lines, each containing two integers Xi and Yi (|Xi|, |Yi| <= 20000). These numbers are the coordinates of the i-th point. When we connect the points in the given order, we get a polygon. You may assume that the edges never touch each other (except the neighboring ones) and that they never cross. The area of the polygon is never zero, i.e. it cannot collapse into a single line.

Output Print exactly one line for each test case. The line should contain exactly two numbers separated by one space. These numbers are the coordinates of the centre of gravity. Round the coordinates to the nearest number with exactly two digits after the decimal point (0.005 rounds up to 0.01). Note that the centre of gravity may be outside the polygon, if its shape is not convex. If there is such a case in the input data, print the centre anyway.

Sample Input

2
4
5 0
0 5
-5 0
0 -5
4
1 1
11 1
11 11
1 11

Sample Output

0.00 0.00
6.00 6.00

Source Central Europe 1999

題意：求多邊形的重心；

意解：
定理1　已知三角形△A1A2A3的頂點坐標Ai ( xi , yi ) ( i =1, 2, 3) 。它的重心坐標為:

　　 xg = (x1+x2+x3) / 3 ; yg = (y1+y2+y3) / 3 ;

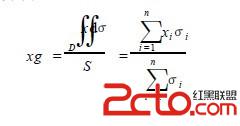
定理2: 將多邊形劃分成n個小三角形區域, 每個小區域面積為σi ,重心為Gi ( . xi , . yi ) ,利用求平面薄板重心公式把積分變
　　 成累加和:

　　　　

AC代碼：

#include 
#include 
#include 

using namespace std;

struct Point
{
    double x,y;
    Point(double x = 0, double y = 0) : x(x),y(y) {};
    void read()
    {
        scanf("%lf %lf",&x,&y);
    }
    Point operator - (const Point &a) const //重載減號；
    {
        return Point(a.x - x, a.y - y);
    }
};

double cross(Point A, Point B) //向量的叉積;
{
    return A.x * B.y - A.y * B.x;
}

double area(Point a, Point b, Point c) //三角型面積公式1/2 * cross(Point A, Point B)
{
    Point A,B;
    A = b - a;
    B = c - a;
    return cross(A,B);//不需要再除以2，因為在最後的結果中抵消了；
}

int main()
{
    int T,n;
    scanf("%d",&T);
    while(T--)
    {
        scanf("%d",&n);
        Point p0,p1,p2;
        double sum_x,sum_y,sum_area;
        p0.read();
        p1.read();
        sum_x = sum_y = sum_area = 0.0;
        for(int i = 2; i < n; i++)
        {
            p2.read();
            double tp = area(p0,p1,p2);
            sum_x += (p0.x + p1.x + p2.x) * tp;
            sum_y += (p0.y + p1.y + p2.y) * tp;
            sum_area += tp;
            p1 = p2;
        }
        printf("%.2lf %.2lf\n",sum_x / sum_area / 3.0, sum_y / sum_area / 3.0);
    }
    return 0;
}