UVA - 11927 Games Are Important (SG)

Description

 

Games Are Important One of the primary hobbies (and research topics!) among Computing Science students at the University of Alberta is, of course, the playing of games. People here like playing games very much, but the problem is that the games may get solved completely--as happened in the case of Checkers. Generalization of games is the only hope, but worries that they will be solved linger still. Here is an example of a generalization of a two player game which can also be solved.

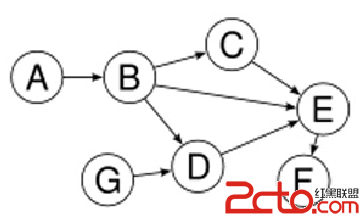
Suppose we have a directed acyclic graph with some number of stones at each node. Two players take turns moving a stone from any node to one of its neighbours, following a directed edge. The player that cannot move any stone loses the game. Note that multiple stones may occupy the same node at any given time.

Input

The input consists of a number of test cases. Each test case begins with a line containing two integers n and m, the number of nodes and the number of edges respectively. ( 1n1000, 0m10000). Then, m lines follow, each containing two integers a and b: the starting and ending node of the edge (nodes are labeled from 0 to n - 1).

The test case is terminated by n more integers s₀,..., s_n-1 (one per line), where s_i represents the number of stones that are initially placed on node i ( 0si1000).

Each test case is followed by a blank line, and input is terminated by a line containing `0 0' which should not be processed.

Output

For each test case output a single line with either the word ` First' if the first player will win, or the word ` Second' if the second player will win (assuming optimal play by both sides).

Sample Input

4 3
0 1
1 2
2 3
1
0
0
0

7 7
0 1
0 2
0 4
2 3
4 5
5 6
4 3
1
0
1
0
1
0
0

0 0

Sample Output

First
Second
有一個DAG(有向五環圖)，每個結點上都有一些石子。兩個玩家輪流把一個石頭從一個結點沿著從此點出發的任意一條有向邊移向相鄰結點。不能移動的玩家算輸掉游戲。注
意，在同一個時刻一個節點上可以有任意的石頭。
思路：注意到，各個石頭的狀態的是完全獨立的，所以這個游戲可以看做每個石頭所形成的游戲的和。對於每一個石頭，它的狀態x就是所在的結點編號，如果此結點已經沒有出發的邊，則既是先手必敗的狀態，否則後續狀態就是相鄰結點的SG值集合。 

需要注意的是，對於在同一個結點來說，其上的石頭如果個數為奇數，則當成1個石頭即可；如果為偶數，可以忽略不計。這是由異或運算的性質決定的。

 

#include 
#include 
#include 
#include 
#include 
using namespace std;
const int maxn = 10005;

int n, m, sg[maxn];
vector g[maxn];

int SG(int u) {
	if (sg[u] != -1)
		return sg[u];

	int vis[maxn];
	memset(vis, 0, sizeof(vis));
	for (int i = 0; i < g[u].size(); i++) {
		int tmp = SG(g[u][i]);
		vis[tmp] = 1;
	}

	for (int j = 0; ; j++)
		if (!vis[j]) {
			sg[u] = j;
			break;
		}
	return sg[u];
}

int main() {	
	int u, v;
	while (scanf("%d%d", &n, &m) != EOF && n+m) {
		memset(sg, -1, sizeof(sg));
		for (int i = 0; i < maxn; i++)
			g[i].clear();

		for (int i = 0; i < m; i++) {
			scanf("%d%d", &u, &v);
			g[u].push_back(v);
		}

		for (int i = 0; i < n; i++)
			sg[i] = SG(i);
	
		int ans = 0, u;
		for (int i = 0; i < n; i++) {
			scanf("%d", &u);
			if (u & 1)
				ans ^= sg[i];
		}
		printf("%s\n", ans ? "First": "Second");
	}
	return 0;
}