規律數組的打印
【北京直真筆試題】打印數組如下4*4數組,要求打印N*N的數組?
1 12 11 10
2 13 16 9
3 14 15 8
4 5 6 7
【思路】:
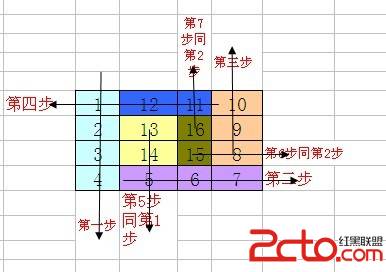
1.發現規律;如上圖所示,仔細發現是有規律的,先第1、2、3、4步驟;我們發現第5、6、7…步驟和前面的1、2、3、4步驟是相同的,只是邊界值不同。
2.考慮實現;實現的問題轉換為先定義二維數組,數組的元素為0.然後的操作就是填值的過程。邊界值的確定采用層層剝離的思想。我們容易看出循環的次數正是N/2次。存在當N為奇數時需要單獨處理最後一個數的情況。
【算法實現】:
//design[思想:層層剝離.]
[cpp]
void designArray(int nArray[][g_nCnt], int nSize)
{
int nBase = 1;
for(int i = 0; i < g_nCnt/2; i++)
{
for(int j = i; j < g_nCnt-i; j++)
{
nArray[i][j] = nBase++;
}
for(int j = i+1; j < g_nCnt-i; j++)
{
nArray[j][g_nCnt-i-1] = nBase++;
}
for(int j = g_nCnt-i-2; j >= i; j--)
{
nArray[g_nCnt-i-1][j] = nBase++;
}
for(int j = g_nCnt-i-2; j > i; j--)
{
nArray[j][i] = nBase++;
}
if(nSize%2 == 1)
{
nArray[nSize/2][nSize/2] = nBase;
}
}//end for i
}
//printArray
void printArray(int nArray[][g_nCnt], int nSize)
{
static int s_nCnt = 0;
cout << "----------------------DESIGN " << ++s_nCnt ;
cout << "----------------------" << endl;
for(int i=0; i <nSize; i++)
{
for(int j =0; j < nSize; j++)
{
cout << nArray[i][j] << "\t";
}//end for j
cout << endl;
}//end for i
cout << "----------------------\\DESIGN " << s_nCnt ;
cout << "----------------------" << endl;
cout << endl << endl;
}
void designArray_t(int nArray[][g_nCnt], int nSize)
{
int nBase = 1;
for(int i = 0; i < g_nCnt/2; i++)
{
for(int j = i; j < g_nCnt-i; j++)
{
nArray[j][i] = nBase++;
}
for(int j = i+1; j < g_nCnt-i; j++)
{
nArray[g_nCnt-i-1][j] = nBase++;
}
for(int j = g_nCnt-i-2; j >= i; j--)
{
nArray[j][g_nCnt-i-1] = nBase++;
}
for(int j = g_nCnt-i-2; j > i; j--)
{
nArray[i][j]= nBase++;
}
if(nSize%2 == 1)
{
nArray[nSize/2][nSize/2] = nBase; //N為奇數,最後元素的處理.
}
}//end for i
}
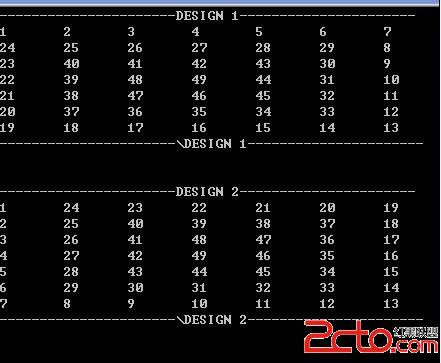
[運行結果]: